
Trapezoids are one of the most interesting and useful quadrilaterals in geometry, and understanding their properties is essential for math students. A trapezoid is a quadrilateral with at least one pair of parallel sides, and it has several unique properties that make it a crucial shape in mathematics and real-life applications.
In this article, we will delve into the properties of trapezoids, their formulas, and provide a comprehensive worksheet for math students to practice and reinforce their understanding of this essential geometric shape.
What is a Trapezoid?
A trapezoid is a quadrilateral with at least one pair of parallel sides. The parallel sides are called the bases, and the non-parallel sides are called the legs. The bases can be of different lengths, and the legs can be of different lengths as well.
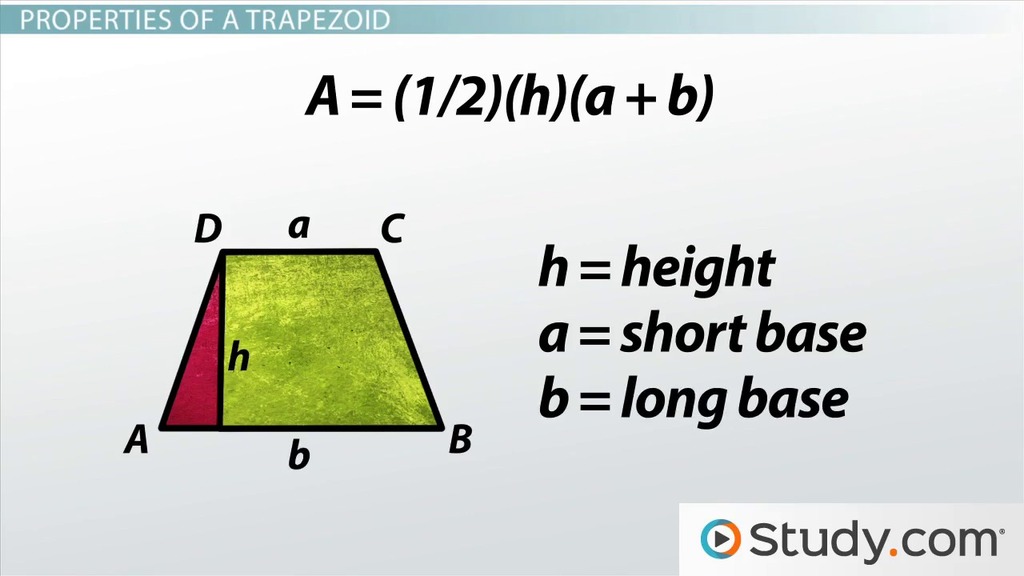
Properties of Trapezoids
Trapezoids have several unique properties that make them essential in geometry and real-life applications. Some of the key properties of trapezoids include:
- The bases are parallel to each other.
- The legs are not parallel to each other.
- The diagonals are not perpendicular to each other.
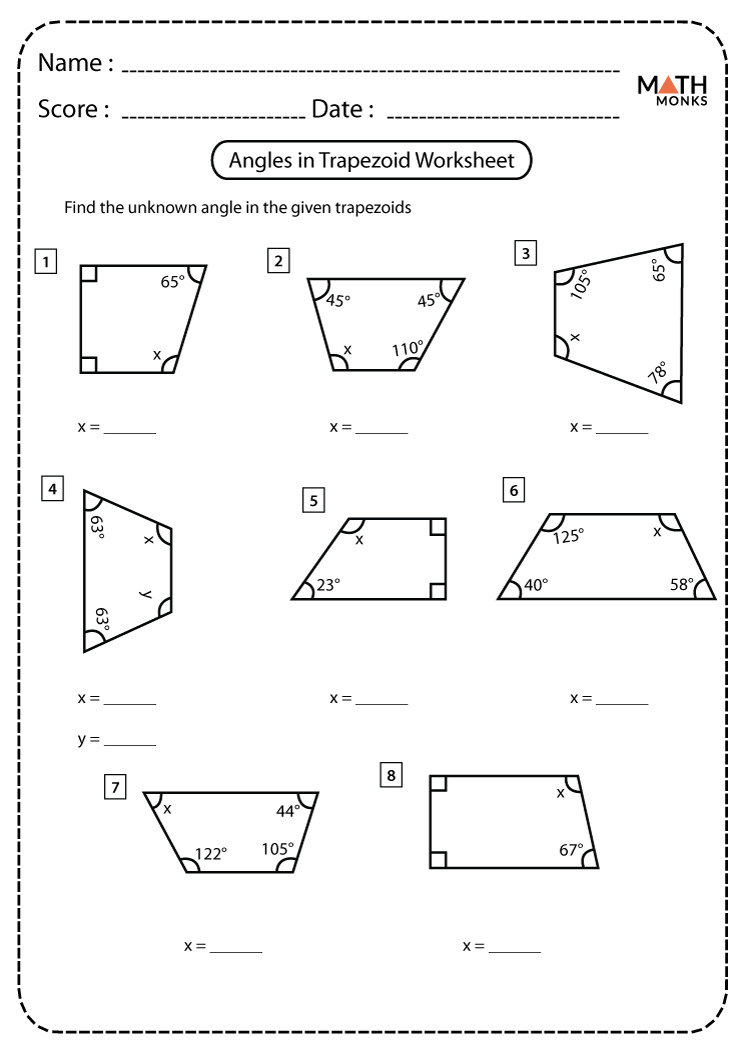
- The sum of the interior angles is 360 degrees.
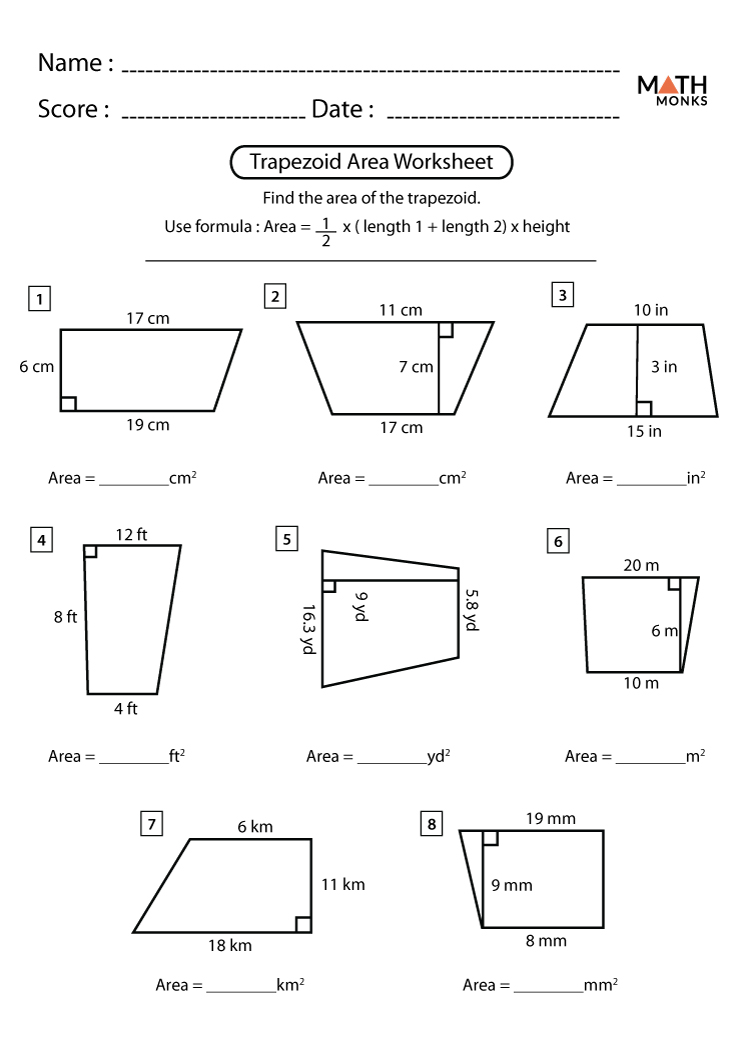
- The area of a trapezoid can be calculated using the formula: A = (1/2) × (a + b) × h, where a and b are the lengths of the bases, and h is the height.
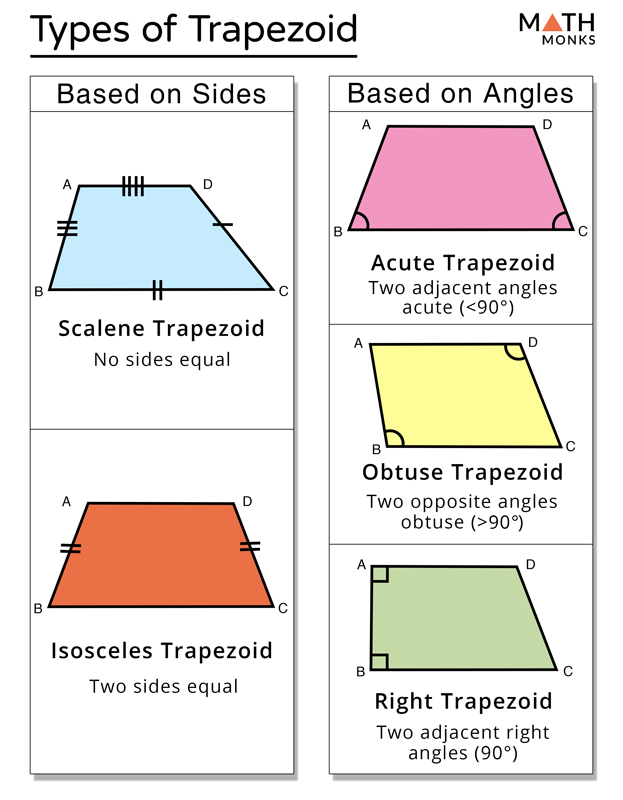
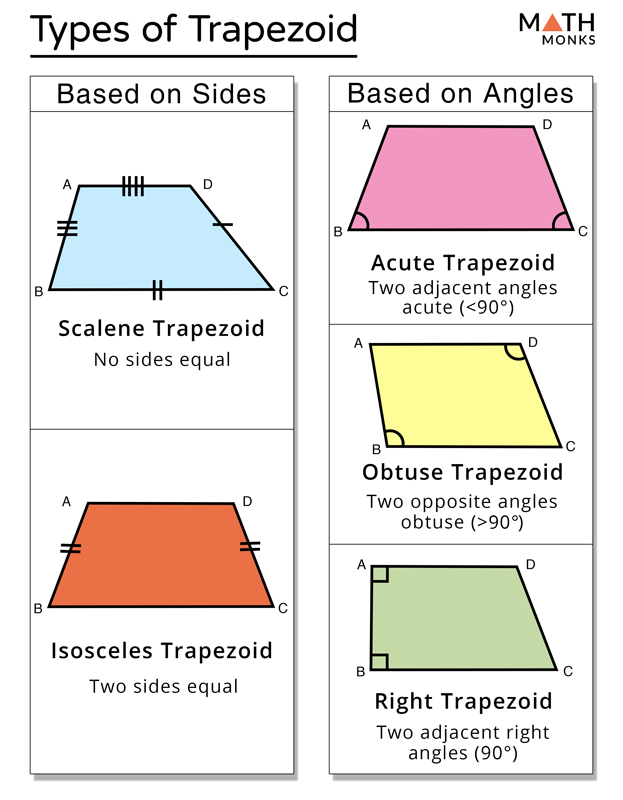
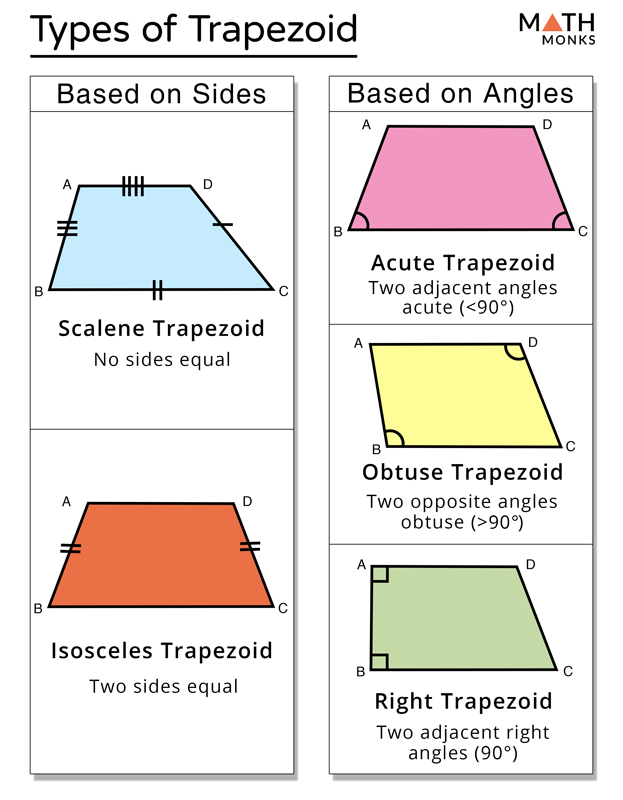
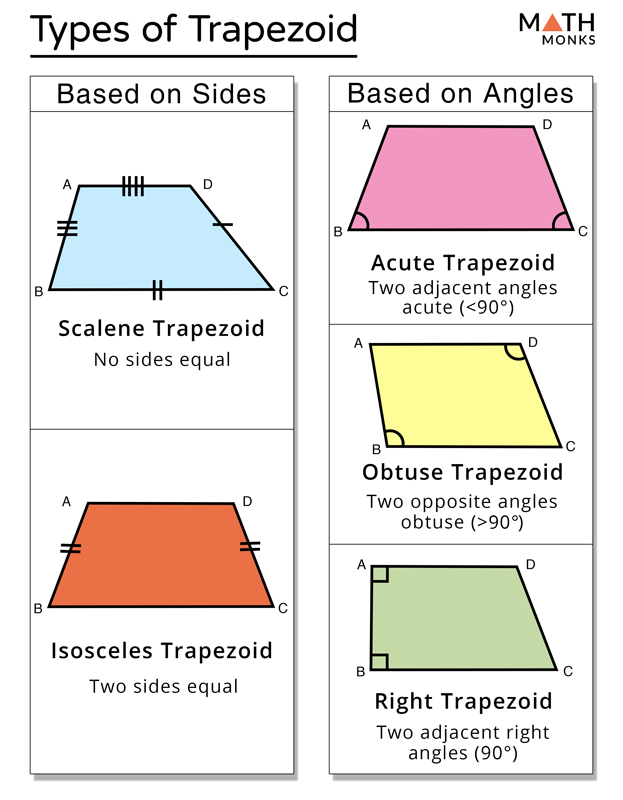
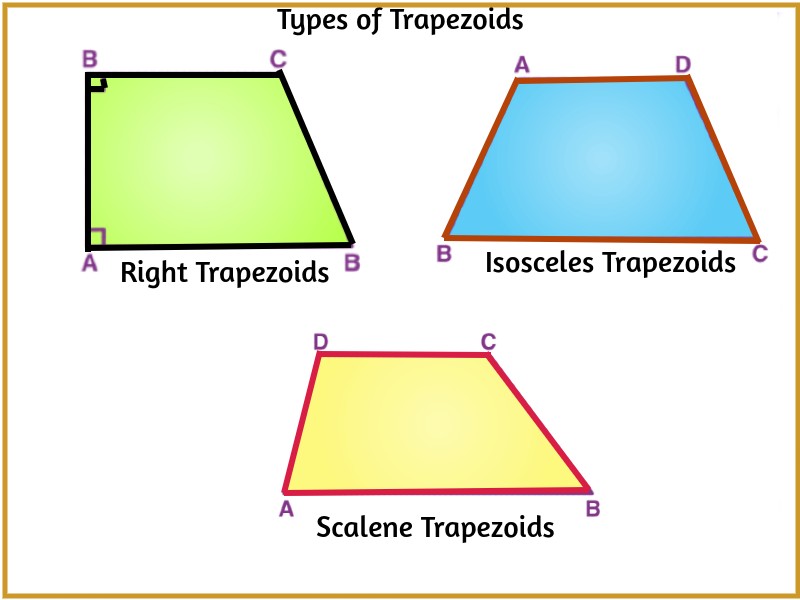
Types of Trapezoids
There are several types of trapezoids, including:
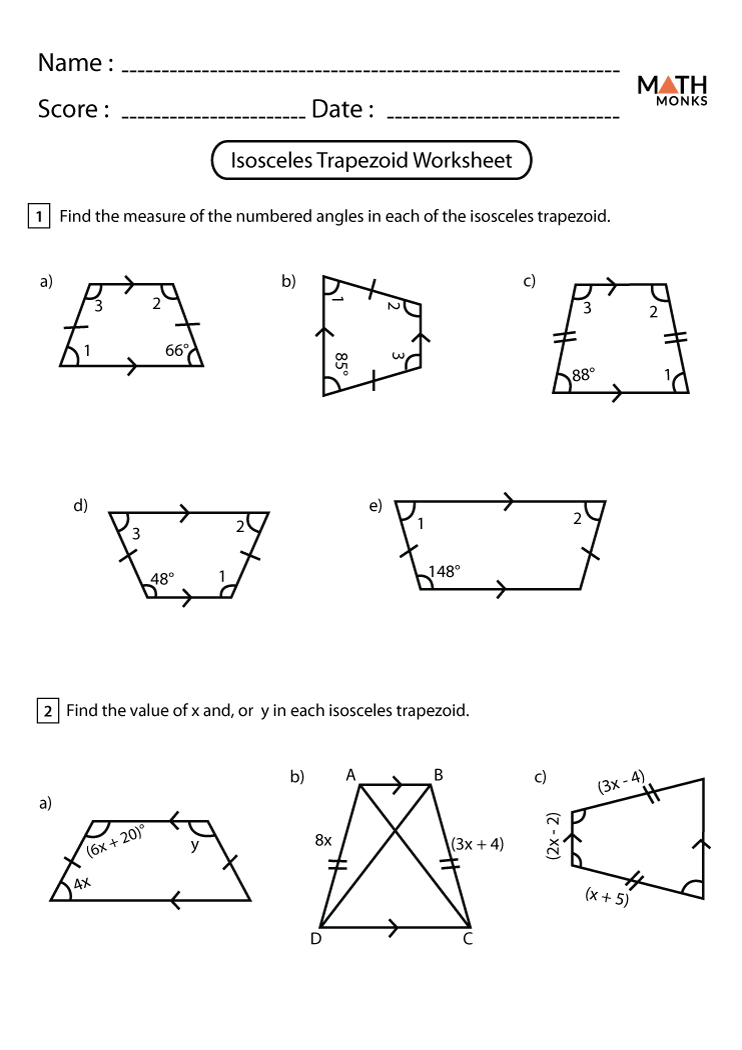
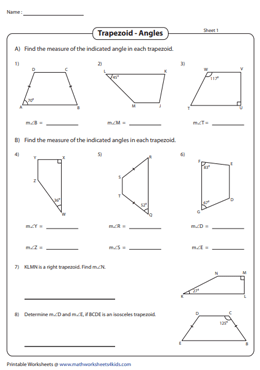
- Isosceles trapezoid: A trapezoid with two legs of equal length.
- Right trapezoid: A trapezoid with two right angles.
- Oblique trapezoid: A trapezoid with no right angles.
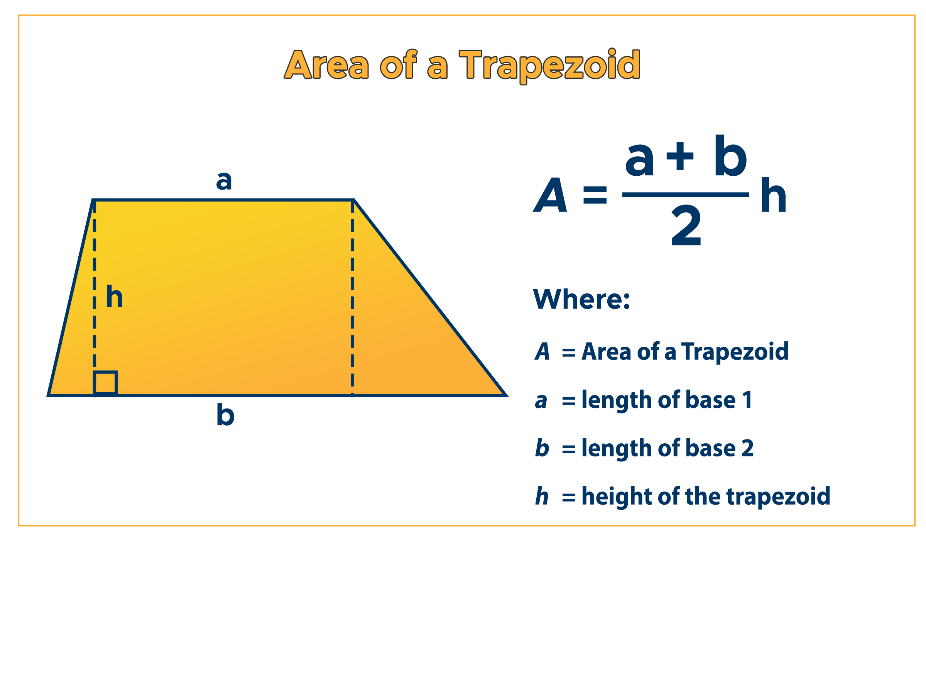
Trapezoid Formulas
There are several formulas that can be used to calculate the area, perimeter, and other properties of trapezoids. Some of the most common formulas include:
- Area: A = (1/2) × (a + b) × h
- Perimeter: P = a + b + c + d
- Height: h = (A × 2) / (a + b)
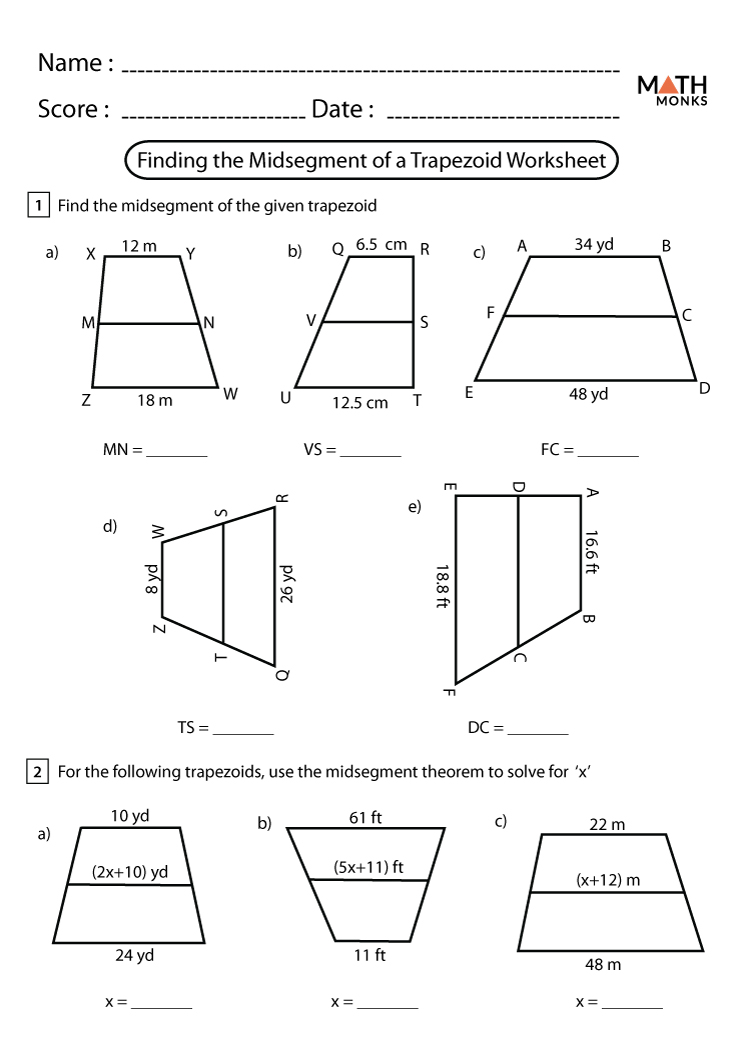
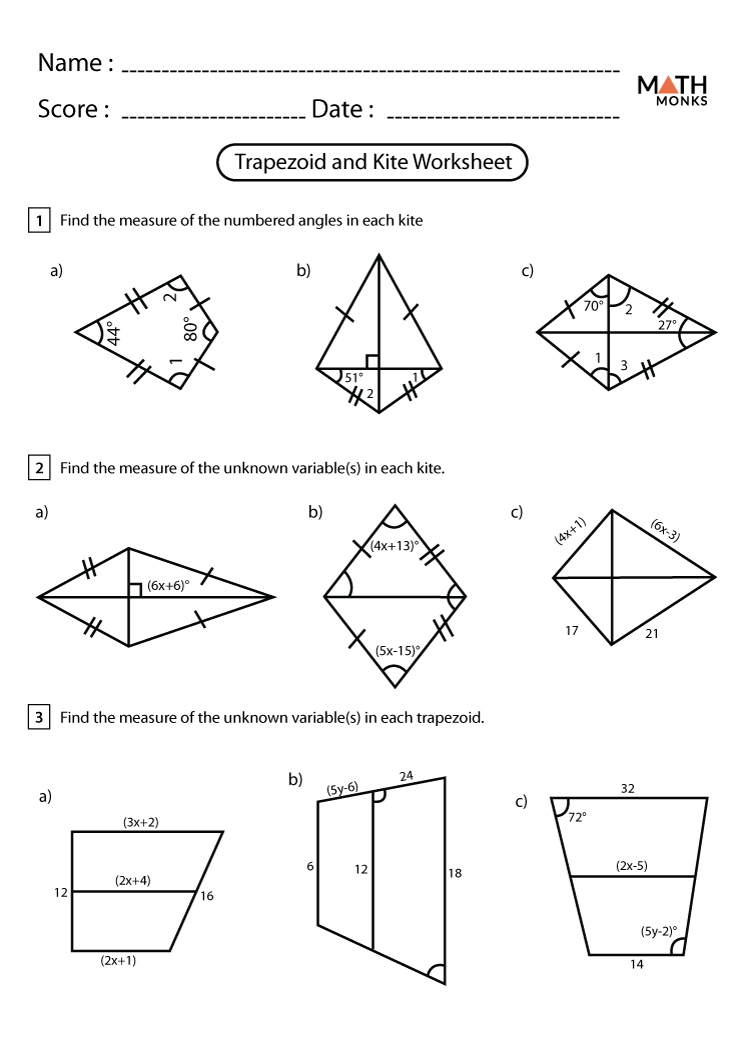
Trapezoid Properties Worksheet
Now that we have explored the properties and formulas of trapezoids, it's time to practice and reinforce your understanding of this essential geometric shape. Here is a comprehensive worksheet with 10 questions to help you practice and apply your knowledge of trapezoids.
- What is the definition of a trapezoid?
- What are the properties of a trapezoid?
- What is the formula for the area of a trapezoid?
- What is the formula for the perimeter of a trapezoid?
- What is the formula for the height of a trapezoid?
- If a trapezoid has two bases of length 5 cm and 10 cm, and a height of 4 cm, what is its area?
- If a trapezoid has two legs of length 6 cm and 8 cm, and a height of 5 cm, what is its perimeter?
- If a trapezoid has an area of 24 cm², and two bases of length 4 cm and 6 cm, what is its height?
- If a trapezoid has a perimeter of 30 cm, and two legs of length 6 cm and 8 cm, what is the length of its third leg?
- If a trapezoid has a height of 6 cm, and two bases of length 5 cm and 10 cm, what is its area?
Gallery of Trapezoid Images
What is the difference between a trapezoid and a parallelogram?
+A trapezoid has at least one pair of parallel sides, while a parallelogram has two pairs of parallel sides.
What is the formula for the area of a trapezoid?
+The formula for the area of a trapezoid is A = (1/2) × (a + b) × h, where a and b are the lengths of the bases, and h is the height.
What is the perimeter of a trapezoid?
+The perimeter of a trapezoid is the sum of the lengths of all its sides, which is P = a + b + c + d.
In conclusion, trapezoids are an essential geometric shape with several unique properties and formulas. By understanding and applying these properties, math students can solve problems and real-life applications involving trapezoids. We hope this article and worksheet have helped you practice and reinforce your understanding of trapezoids.




![Free Area Of A Trapezoid Worksheets for Kids [PDFs] Brighterly](https://brighterly.com/wp-content/uploads/2022/10/area-of-a-trapezoid-worksheets-images-4.jpg)
