5 Negative Number Equation Sheets To Practice

Mastering negative number equations is a crucial step in developing strong math skills, particularly in algebra and beyond. Understanding how to work with negative numbers can seem daunting at first, but with practice, it becomes more manageable. Here, we'll explore the concept of negative number equations and provide you with resources to practice.

Negative numbers are a fundamental concept in mathematics, representing a quantity that is less than zero. When dealing with negative numbers in equations, it's essential to remember the rules of operation:

• When adding two negative numbers, you add their absolute values and keep the negative sign.
• When subtracting a negative number, you add its absolute value.
• When multiplying or dividing two negative numbers, the result is positive.
• When multiplying or dividing a negative number by a positive number, the result is negative.

Understanding Negative Number Equations

Negative number equations can involve simple operations like addition, subtraction, multiplication, and division, but they can also be part of more complex equations involving variables. Solving these equations requires careful attention to the signs of the numbers involved.

Simple Negative Number Equations

Let's start with some simple examples to illustrate how negative number equations work:

1. -3 + (-5) =?
2. -2 - (-4) =?
3. -6 * (-3) =?
4. -10 / (-2) =?

Solving these equations involves applying the rules mentioned above.

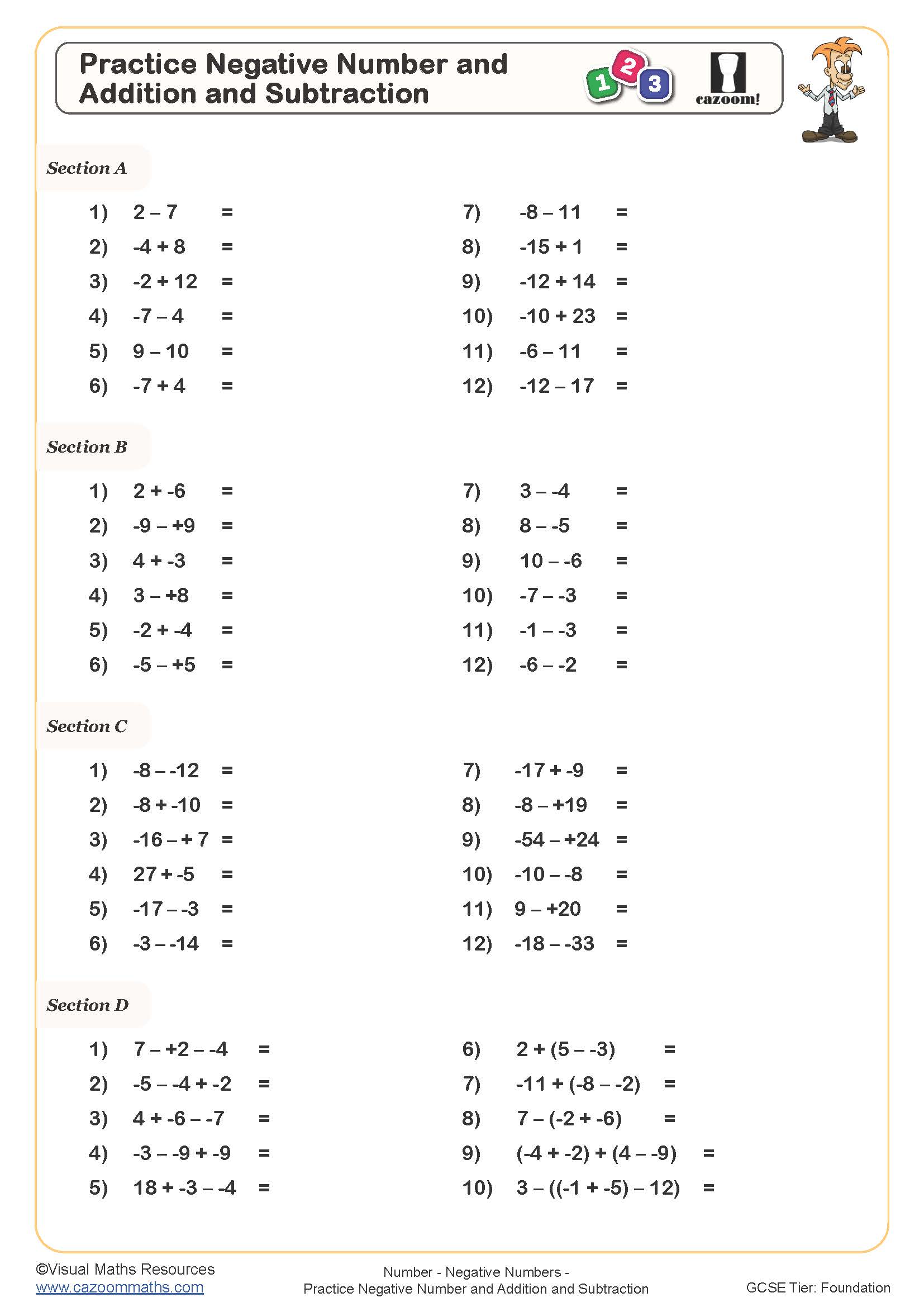
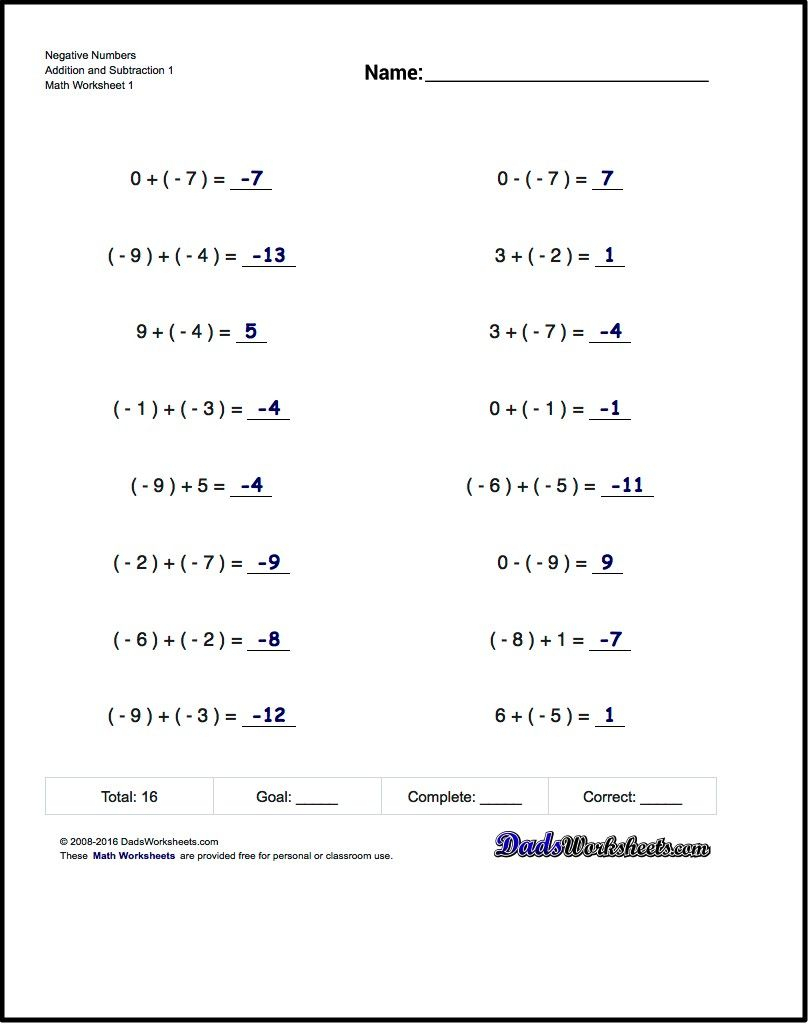
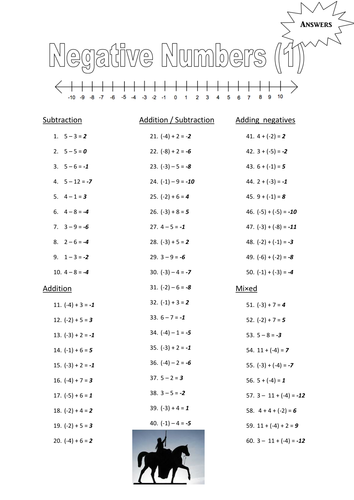
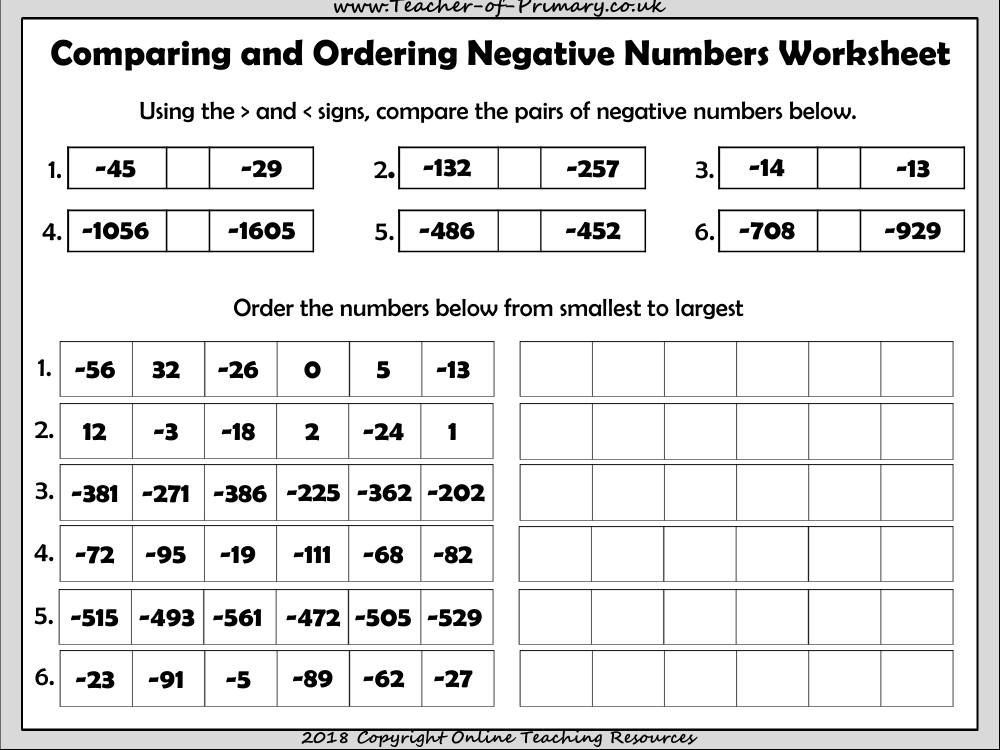
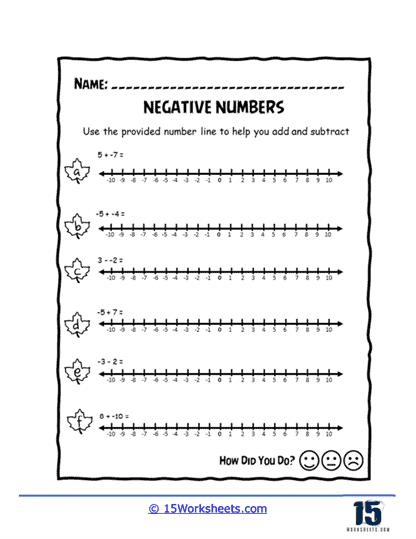
Practice Sheets for Negative Number Equations

To help you practice and reinforce your understanding of negative number equations, here are five practice sheets you can use:

Practice Sheet 1: Basic Operations with Negative Numbers

1. -2 + (-5) =
2. -7 - (-3) =
3. -9 * (-1) =
4. -12 / (-4) =
5. -15 + (-2) =

Practice Sheet 2: Simplifying Expressions with Negative Numbers

1. Simplify: -3 * (-2) + (-5)
2. Simplify: -10 / (-2) - (-3)
3. Simplify: -7 - (-9) + (-1)
4. Simplify: -15 * (-3) / (-5)
5. Simplify: -20 + (-8) - (-12)

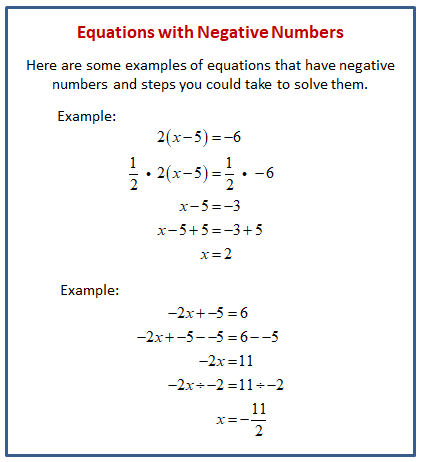
Practice Sheet 3: Equations with Variables and Negative Numbers

1. Solve for x: x - 3 = -5
2. Solve for y: 2y + 5 = -3
3. Solve for z: z / -2 = 4
4. Solve for w: w - 2 = -1
5. Solve for v: v + 1 = -9

Practice Sheet 4: Mixed Operations with Negative Numbers

1. Simplify: -2 + (-5) * (-3)
2. Simplify: -10 / (-2) + (-1)
3. Simplify: -7 - (-9) / (-3)
4. Simplify: -15 * (-3) + (-5)
5. Simplify: -20 + (-8) * (-2)

Practice Sheet 5: Real-World Applications of Negative Number Equations

1. Temperature Change: The temperature outside was -5°C. It dropped by 3°C. What is the new temperature?
2. Financial Transactions: If you have -$20 in your account and deposit $15, what is your new balance?
3. Height Below Sea Level: A cave is 20 meters below sea level. If you descend another 8 meters, how far below sea level are you?
4. Investment Loss: An investment lost $500 one day and another $200 the next. What is the total loss?
5. Depth of a Hole: A hole is 15 meters deep. If it is dug 3 meters deeper, how deep is it now?

Conclusion: Mastering Negative Number Equations

Mastering negative number equations is a skill that requires practice and patience. By working through the practice sheets provided, you can improve your understanding and confidence in dealing with negative numbers in various mathematical contexts. Remember, practice is key to reinforcing your knowledge and becoming proficient in solving negative number equations.

Gallery of 5 Negative Number Equation Sheets To Practice